There are 4 red marbles 3 yellow 2 green and 1 blue marble.
Is picking two marbles permutation.
So if we have 3 tin cans to give away there are 3.
For permutations without repetition we need to reduce the number of objects that we can choose from the set each time.
Fancy word for just a simple idea that the sample.
Two marbles are drawn without replacement.
If we want to figure out how many combinations we have we just create all the permutations and divide by all the redundancies.
A draw the tree diagram for the experiment.
There s two green marbles in the bag.
Endgroup jessica oct 6 13 at 3 36.
B find probabilities for p bb p br p rb p ww p at least one red p exactly one red 3.
Algebra permutations solution.
Why do the two solutions differ.
It is crucial that you are choosing items without replacement for the two methods to be equivalent.
Or 6 variations for every choice we pick.
So i could pick that green marble or that green marble.
A bag of marbles containing 4 white marbles and 6 red marbles.
And sometimes this is referred to as the sample space the set of all the possible outcomes.
So this is all the possible outcomes.
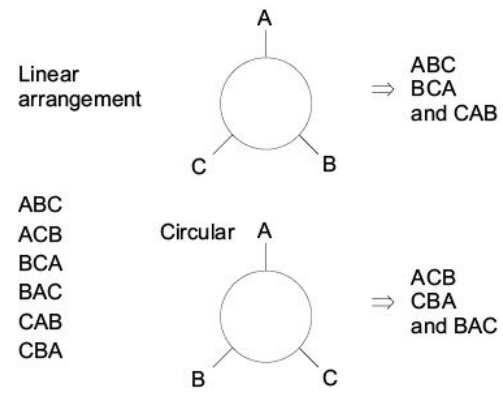
Choosing a first marble then a second is the same as first choosing two marbles then picking which goes first.
If we randomly select two marbles from the bag what is the probability that the selected marbles are of different colors in other words one white and one red.
1 after marble no 2 is different from vice versa.
Assuming each color of marble is identical and it doesn t matter which specific marble of each color is chosen calculate the number of possible permutations in which you can remove the 10 marbles.
In this case you know whether both are red after the first step so the second step is not necessary and you can work with combinations.
Endgroup sudarsan oct 6 13 at 3 24 begingroup assuming the marbles are identical and order doesn t matter.
There s one blue marble.
For example given that we have 5 different colored marbles blue green red yellow and purple if we choose 2 marbles at a time once we pick the blue marble the next marble cannot be blue.
Suppose an opaque jar contains 4 red marbles and 10 green marbles the following exercise refers to the experiment of picking two marbles from the jar without replacing the first o log on.
Selecting k objects from n objects is given by.
In our case we get 336 permutations from above and we divide by the 6 redundancies for each permutation and get 336 6 56.
Two marbles are drawn without replacement from a jar containing 4 black and 6 white marbles.